Re: considering the diameter of the sun what is the different effect of light h

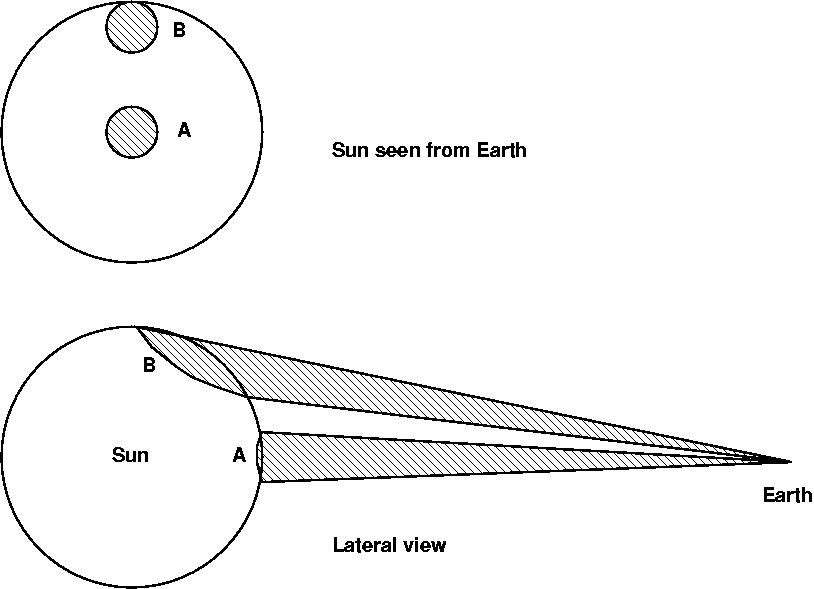
The amount of energy in a beam of light decreases with distance. The decrease is proportional to the square of the distance. That is to say that if a point in space is twice as far from a luminous source than another point, the first point will receive four times less energy than the second point. However we also have to worry about the way that the luminous source emits light. From Earth we see more surface of the poles of the Sun than that of the equator of the Sun. Therefore the decrease of energy with distance is compensated. Let me try to explain this. Imagine that we see only a small part of the solar disk through a circular hole in a cardboard. (DO NOT TRY THIS IN REAL LIFE. THIS IS AN IMAGINARY EXPERIMENT. LOOKING DIRECTLY AT THE SUN CAN CAUSE BLINDNESS.) If we see a part of the solar disk near its center, the light coming through the hole will be light emitted from a sector on the surface of the Sun, which I have labeled "A" in the figure below. That light travels to Earth from all points of sector "A" through a cone, which I have shown as a shaded kind of triangle ending on Earth in the lower part of the figure. If we now move the cardboard to see a part of the solar disk near its edge, the light coming through the hole will be emitted from sector B of the surface. The angle of both cones at Earth is the same, but the surface of sector B is larger than the surface of sector A. Thus sector A contributes less energy than sector B to the cone because it has a smaller area than sector B. But the energy coming from sector B decreases more than the energy coming from sector A because it is farther from Earth. Which effect is more important, the larger surface of sector B, or the larger distance of sector B with respect to sector A? If every point of the surface of the Sun were equally bright, both effects would compensate each other exactly. One can demonstrate mathematically using calculus that sector A and sector B contribute equally to the energy arriving at Earth. If you want to know how to do it, I recommend reading chapter 1 of a graduate text: Radiative Processes in Astrophysics by Rybicki and Lightman.

The solar surface is not equally bright anyways. There are sunspots and flares. Therefore there is no need to worry so much about calculus here. The distance effect is really not that important. To begin with, I placed Earth very close to the Sun in the above diagram. In reality Earth is 215 times farther from the Sun than the Sun's radius. Thus brightness differences are not so important here. Let us put some numbers. The Earth-Sun distance is about 92.9 million miles. The solar radius is 432,000 miles. That is the difference in distance to Earth between the pole and the equator of the Sun. What difference does it make 432,000 miles in 92.9 million miles, taking into account the variations of the light energy with the square of the distance? We can use some algebra here. If I call d the distance to the pole, and I call r the radius of the Sun, the difference in energy from the equator minus the energy from the pole is proportional to
1/(d-r)²-1/d². That difference represents an increase of 0.94 percent.

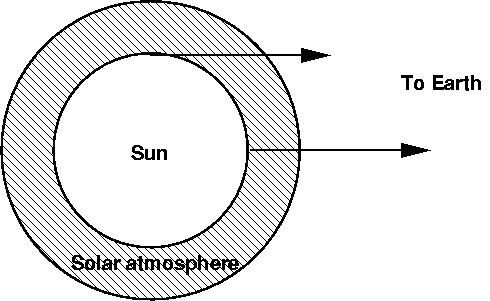
In reality the edges of the solar disk do look dimmer than its center, not because they are farther, but because of the Sun's atmosphere. The Sun has a thin atmosphere over its surface, shown as a shaded ring on the left in the figure below (I exaggerated the thickness of the solar atmosphere in the figure for reasons of clarity). Therefore light rays leaving its surface near the edges of the solar disk towards the Earth have to traverse a larger mass of solar atmosphere than rays leaving the solar surface from points near the center of the solar disk as shown in the figure. Thus one expects a dimmer light coming from the edges of the solar disk. This effect is called "limb darkening" as shown in the photograph on the right. (The dark dot to the lower right of the center is planet Mercury crossing the solar disk on November 6, 2008, and the bigger dark spot near the left edge is a sunspot. See a bigger version of this beautiful photograph in the Wikimedia Commons.)

Explanation of Limb Darkening		Photograph by Mila Zinkova reproduced here under the Creative Commons Attribution ShareAlike 3.0 License

Greetings,

Vladimir Escalante-Ramirez