Re: Does sound travel in a cone or sphere?

Hi Graham,

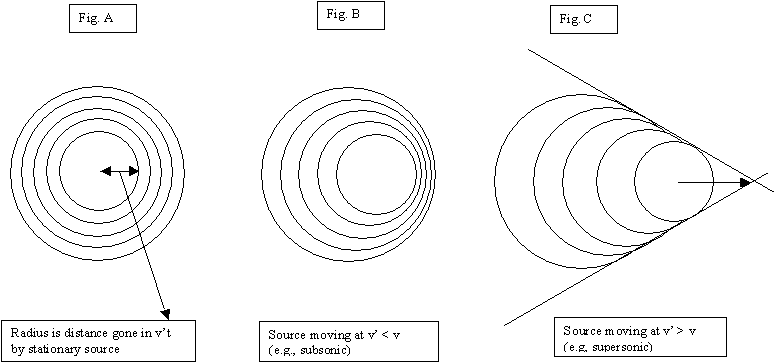
Okay, there are two approaches to your question. The short answer is that sound waves are normally spherical waves that radiate outward from their source. Fig. A below shows spherical sound waves represented in 2 dimensions (a sphere is simply a circle in 2-d). Okay, that's the short answer but that is only true for stationary sources (that is, the source that emits the sound isn't moving). Btw, in all this, we'll also assume that the observer (the person hearing the sounds) is also stationary.

Since we'll be referring to the figures frequently, let's go over Fig. A briefly. You can look at it in 2 ways. The first way is to think of Fig. A as showing us the waves propagating in space, where each subsequent circle represents a different wave. The other is to look at it as a wave propagating in time, where all the circles represent the same wave at different times. We'll favour the first approach here (so each circle is a different wave, emitted at a different time; thus, wave 5 (the largest, outermost circle) in Fig. A was emitted first and has gone the farthest while wave 1 (the smallest, innermost circle) in Fig. A was just emitted and is just starting to spread out).

Now the situation gets more interesting when the source starts to move, with respect to the observer. Fig. A shows the propagation of the sound waves after they're emitted by the source. The latest wave (the innermost circle, circle 1) has only managed to go out a distance v*t while the biggest circle, the first wave that was emitted (the outermost circle, circle 5) has gone out a much longer distance (say, v*(t+t(0))). As long as everything is stationary, these waves just continue to expand spherically, each taking the place of the earlier wave after it's moved on. But, as the source starts to move (at a velocity, v'), the newly emitted waves start to catch up with the previously emitted waves. As a result, the waves start to bunch up, as shown in Fig. B; wavelengths become shorter and frequencies become higher. This is, of course, another instance of the Doppler effect, which you might have heard about in class (if your class hasn't discussed it yet, you might want to go to your local library and ask the librarian for an introductory book on physics that covers it).

Now, as the source starts to move faster and faster, things start to get even more interesting. The waves tend to travel in their medium at a certain speed of propagation, the phase speed of the wave in that medium, called v in the figures. Just as water waves spread out at a certain speed, sound waves also spread out at a certain speed. But, as the source starts to approach and pass that speed of wave propagation (i.e., v' becomes greater than v), the regular Doppler shift formulas have to be modified. This is because as the source moves at supersonic speeds (i.e., faster than the speed of sound in "normal" air), the newly emitted waves actually start to catch up and pass the previously emitted wave. Looking at Fig. C, let's once again think of the biggest circle as the first wave that was emitted. As that wave spreads out at it's phase speed, the source continues to move forward faster than the wave.

Then, when the source emits a new wave, that wave spreads out at the phase speed of the wave. But since it was emitted when the source had already moved forward relative to the previous wave, it (the new wave) is farther than it would have been if the source hadn't been moving, thus eventually overlapping with the previous wave. And this process continues as long as the source keeps moving faster than the wave propagation velocity. The edges of the wave overlaps (the envelope) forms a cone shape and, for sound waves, this cone is called the Mach cone and any aircraft that travels at supersonic speeds has one (it actually has two: one from the front part and one from the tail part). In fact, the so-called sonic boom that you hear is a result of this; it's not so much that the aircraft has broken the "sound-barrier" but rather the buildup of the radiant sound energy in one spot (that spot being the intersection of the mach cone with the observer, i.e., you). And these sort of envelopes are features of all waves in their respective mediums (e.g, a fast moving boat on water also has this "cone", in this case the bow wave). So, to summarize the (really) long answer, sound usually travels in spherical wavefronts but, under certain conditions, can also "travel in cone form". I hope that helped!

Best regards,

Rick.