Date: Wed Oct 11 17:51:29 2000
Posted By: Steven Miller, Undergraduate, Mechanical Engineering, San Diego State University
Area of science: Engineering
ID: 968196040.Eg
Message:
William,
This is quite a bag of worms you have opened up! Bicycle frame
geometry and its effect on handling seems to be very subjective issue. We
can, however, still discuss the fundamental mechanics/geometry of the
steering portion of the problem and see how this may effect the operator’s
perception of stability.
When you say caster I believe you are referring to what is called
headtube angle (HTA), which is an angle formed by a horizontal plane (the
ground) and the centerline of the headtube extended to intersect the
ground. The angle is measured so that the inside of the angle faces the
rear of the bike. For most conventional bicycles this angle is acute
(less than 90 degrees). Most manufacturers of road type machines are
using headtube angles in the 70 -75 degree range as an approximation.
These specifications are readily available from the manufacturers’
websites.
Another concept that is important to steering geometry is that
of “trail”. For our purposes we will define trail as the distance from
where the centerline of the headtube intersects the ground to where a line
drawn through the center of the front axle perpendicular to the ground
intersects the ground. Increased trail changes the characteristics of how
the front wheel responds to steering inputs. For an HTA of 90 degrees,
steering inputs rotate the front wheel in the x-z plane. (x= forward, y=
up, z= to the sides) For an HTA of 0 degrees steering inputs would rotate
the front wheel in the y-z plane. (Note that the centerline of the front
axle lags the centerline of the headtube’s intersection point with the
ground (hence the term trail? It's a good way to remember it, anyway).
Finally there is “rake”, which is measured by drawing a line
perpendicular to the headtube centerline through the center of the front
axle, and measuring from where the two lines intersect to the center of
the axle. For a headtube angle of 90 degrees, the rake and the trail
would be equivalent. Increasing rake slows steering response but improves
shock absorption (a higher proportion of the column works as a cantilever
beam as both rake increases and headtube angle decreases - the material if
not completely rigid then acts to damp impact through flex of the forks).
Basically the concept of stability with respect to steering geometry
as defined above is determined as follows - The higher the trail value,
the “quicker” the steering response and the more stable the bike will feel
at high speeds (less input required to maintain stability - remember that
bicycling is a very dynamic mechanical activity. When pedaling there are
unbalanced torque inputs at the cranks and simultaneous steering
corrections at the bars to compensate. Adding the rider to the system
complicates it more because now the rider can use body position to alter
the center of gravity as required as well.). Lower trail values equate
with better low speed stability and a more “mellow” response to steering
inputs. Basically the increased trail is more conducive to the nature of
turning a single track vehicle at high speeds - we "roll" into directional
changes as opposed to turning the wheels as in a car.
All of this is strictly relative to the bicycle’s design, be it a
mountain, BMX, or road bike - a tandem or perhaps even a recumbant. There
is no hard and fast set of equations to determine what a "good" head tube
angle is. If you design and build a frame and it doesn’t meet your
expectations, changes can be made to the headtube angle to alter the trail
and the handling. The handling of a bike is due to the entire frame
geometry however, not just that of the headtube angle /trail.
Additionally, rigidity of the frame contributes to handling as well (more
rigid is generally more stable and more responsive to inputs.
I won't walk you through any relational equations because they are all
basic geometry - draw the frame, fork and front wheel, extend headtube
lines and draw a line perpendicular with the ground through the headtube
and measure trail. Do this and you can establish the following equation
relating rake, trail and headtube angle (HTA):
Trail= [(tire radius*cos(HTA))-rake]/tan(HTA)
OK, I guess I will walk you through it after all in the following image:
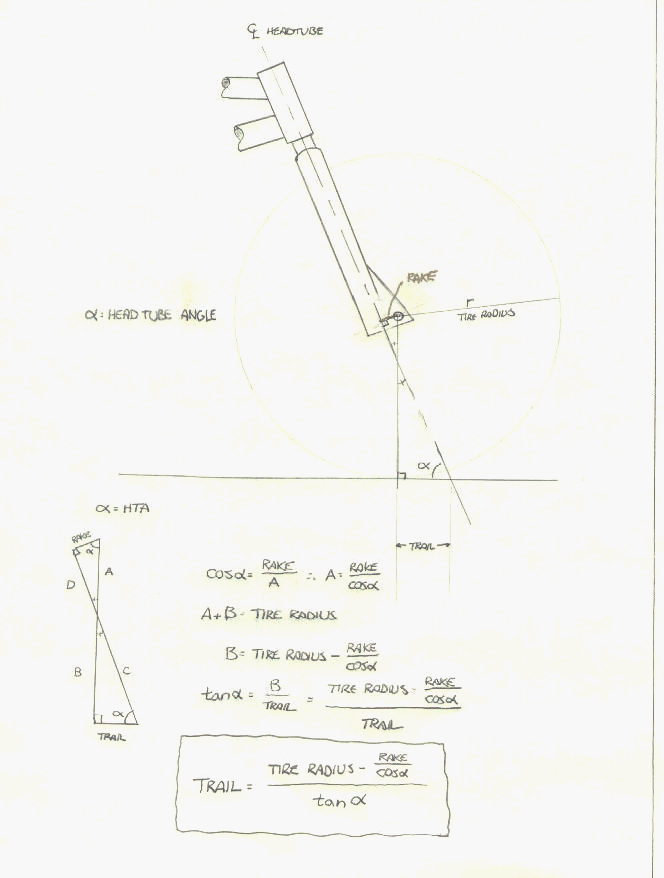 My equation differs from one offered by a manufacturer, however I'm going
to stand by mine as I did it twice and it sure looks right. Check it out
and do it yourself just to be sure. (make sure to do calculations in
radians).
Remember, smaller headtube angle = slower steering response, better low
speed stability and better shock absorption (assuming the forks are solid
forks - for "suspended" forks with springs oil etc. this would not be
true). Just think Easy Rider vs. Kawasaki Ninja. Smaller headtube angle
= larger trail. Good luck.
Sincerely,
Steven Miller
smiller@kahuna.sdsu.edu
Undergrad - Mechanical Engineering
San Diego State University
My equation differs from one offered by a manufacturer, however I'm going
to stand by mine as I did it twice and it sure looks right. Check it out
and do it yourself just to be sure. (make sure to do calculations in
radians).
Remember, smaller headtube angle = slower steering response, better low
speed stability and better shock absorption (assuming the forks are solid
forks - for "suspended" forks with springs oil etc. this would not be
true). Just think Easy Rider vs. Kawasaki Ninja. Smaller headtube angle
= larger trail. Good luck.
Sincerely,
Steven Miller
smiller@kahuna.sdsu.edu
Undergrad - Mechanical Engineering
San Diego State University
Current Queue |
Current Queue for Engineering |
Engineering archives
Try the links in the MadSci Library for more information on Engineering.
MadSci Home | Information |
Search |
Random Knowledge Generator |
MadSci Archives |
Mad Library | MAD Labs |
MAD FAQs |
Ask a ? |
Join Us! |
Help Support MadSci
MadSci Network,
webadmin@www.madsci.org
© 1995-2000. All rights reserved.
 My equation differs from one offered by a manufacturer, however I'm going
to stand by mine as I did it twice and it sure looks right. Check it out
and do it yourself just to be sure. (make sure to do calculations in
radians).
Remember, smaller headtube angle = slower steering response, better low
speed stability and better shock absorption (assuming the forks are solid
forks - for "suspended" forks with springs oil etc. this would not be
true). Just think Easy Rider vs. Kawasaki Ninja. Smaller headtube angle
= larger trail. Good luck.
Sincerely,
Steven Miller
smiller@kahuna.sdsu.edu
Undergrad - Mechanical Engineering
San Diego State University
My equation differs from one offered by a manufacturer, however I'm going
to stand by mine as I did it twice and it sure looks right. Check it out
and do it yourself just to be sure. (make sure to do calculations in
radians).
Remember, smaller headtube angle = slower steering response, better low
speed stability and better shock absorption (assuming the forks are solid
forks - for "suspended" forks with springs oil etc. this would not be
true). Just think Easy Rider vs. Kawasaki Ninja. Smaller headtube angle
= larger trail. Good luck.
Sincerely,
Steven Miller
smiller@kahuna.sdsu.edu
Undergrad - Mechanical Engineering
San Diego State University